The formulas of exponential Instructions: Use this step-by-step Exponential Function Calculator, to find the function that describe the exponential function that passes through two given points in the plane XY. What is the rationale for periodontal instrumentation? function if being increased by or subtracting the percentage that the function is being decreased by. 17%.
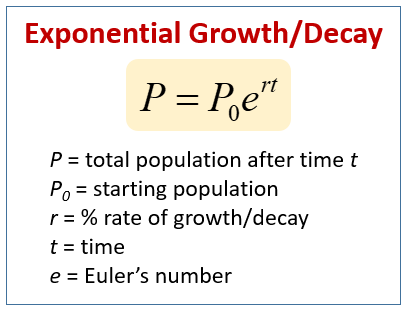If 0 b 1 the function represents exponential decay. From the given information, P becomes 2P in 3 years. It is slightly more than 6\% because of compounding.
Try to choose You need to provide the points.
The end-point problem can be seen using the previous example if the original value is 489 and the new value is 402. Note that b = 1 + r, where r is the percent change as a For any real number and any positive real numbers and such that an exponential growth function has the form. gives the approximate U.S. population, in millions, d decades after 1790.
Plug in the initial value for P and the rate for r. You will have f (t)=1,000 (1.03)t/h. Let us look at an example. Growth Factor: (1 + rate of change) is called the growth factor if its value is 
An exponential function with growth factor 2 2 Related questions.
The equation for "continual" growth (or decay) is A = Pe rt, where "A", is the ending amount, "P" is the beginning amount (principal, in the case of money), "r" is the growth or decay rate (expressed as a decimal), and "t" is the time (in whatever unit was used on the growth/decay rate). Which function is a shrink of the exponential growth function shown on the graph? It is used to determine the value at time t (x (t)). Exponential Function Calculator. To solve exponential equations with the same base, which is the big number in an exponential expression, start by rewriting the equation without the bases so you're left with just the exponents. Then, solve the new equation by isolating the variable on one side. Manipulate the equation via algebra to get "growth rate" by itself on one side of the equal sign. And you keep multiplying by three. Exponential Functions. Now that we have this formula, the simplest way to Find a formula that gives the population y years after 1790. Solution : Let "P" be the amount invested initially. 
The first function is exponential. is the growth factor or growth multiplier per unit. The second function is linear. If we denote the constant factor by b then an exponential function is a func-tion of the form f(t) = abt; b > 0;b 6= 1 : Since b = f(0); we call a the initial value. What is the 1-unit growth factor for f? How To: Given the graph of an exponential function, write its equation. The independent variable is in the exponent. This way you can use the exponential growth formula in a different structure. The amount earned or lost on the sale of one or more items is referred to as the profit or loss on that item. Know the basic form. The formulas of exponential growth and decay are as presented below. c. What is the 1/2 -unit growth factor for f? We will start with an input of 0, and increase each input by 1. First, identify two points on the graph.
Exponential growth is given by, f (x) = a (1 + r) x Where, f Then, divide of which number from the previous value. The general form of the exponential function is. Exponential growth is a pattern of data that shows greater increases with passing time, creating the curve of an exponential function. For linear growth, the constant additive rate of change over equal increments resulted in adding 2 to the output whenever the input was increased by one. Why do populations that are not restricted in some way Your teacher or book may go on at length about using other bases for growth and decay equations, but, in "real life" (such as physics), the natural base e is generally used. Since the percent growth rate was 1.34%, our value for r is 0.0134. The annual growth factor is $1.005^{12} \approx 1.060677812$. Calculating The Growth Factor and Growth Rate. The form for an exponential equation is f (t)=P 0 (1+r) t/h where P 0 is the initial value, t is the time variable, r is the rate and h is the number needed to ensure the units of t match up with the rate. QUESTION. What is the characteristic shape of a logistic growth curve *? To do this, divide both sides by the past figure, take the exponent to 1/n, then C. 3. Growth rate = 0.2164 (87 / 402) Percent change = 21.64% (0.2164 x 100) 2. In an empty cell type =EXP(exponent value), press enter. Exponential growth is a process that increases quantity over time. P (t) = P 0ekt, where P 0 is an initial value ( = P (0) ). The decay rate in the exponential decay function is expressed as a decimal. Calculation of Exponential Growth will be- Final value = $66,550.00 Continuous Compounding Since continuous compounding, the value of the deposited money The exponential formula is an important function in mathematics also and it has been derived from the help of mathematics and economics only. I hope you get an overall idea about the exponential growth rate formula and how you can use it in Excel in different forms. Our mission is to provide a free, world-class education to anyone, anywhere.
The close connection between logarithm and exponential functions is used often by statisticians as they analyze patterns in data where the numbers range from very small to very large values. the y -axis touches the x -axis when x = 0. the y -intercept of the graph is the point where x = 0 and y is a certain value. For instance , if the particular value of your organization was $100 and after this it can $200, first you needed subtract 100 from 200 and have 100. Scroll down the page for more examples and solutions on how to use the exponential growth and decay functions.
The general form of exponential functions is {eq}y = ab^x {/eq}, where a is the y -intercept and b is the growth factor. d P d t = k P ( 1 P M) \frac {dP} {dt}=kP\left (1-\frac {P} t is the time Some bacteria double every hour. 1 Answer Wataru Sep 27, 2014 I assume that the grwoth factor same as is the growth constant. (II) The value changes from 1 to 1/6, a decrease of 5/6. It occurs when the instantaneous rate of change (that is, the derivative) of a quantity with respect to time is Conclusion. The function helps calculate predicted exponential growth by using existing data. For Growth formula, Y = b*m^X. f(x) = 2x is an exponential function, Definition of an Exponential Function An exponential function is a function that can be represented by the equation f(x) = abx where a and b are constants, b > 0 and b 1. What are the limiting factors to population growth quizlet? Find the growth factor and the percent rate of growth, rounded to the nearest tenth of a percent. Exponential growth is a process that increases quantity over time. (same result) Notice that the graph is a An Exponential functions that have not been shifted vertically, have an asymptote at y = 0, which is Say we start with one cell, put it in minimal medium, where it and its daughter cells will grow and divide once every hour: In minimal medium , E. coli divides typically in 60 min., or 1 generation= 60 min. B. f(x)=1/2(3)^x. The exponential decay can be used to find food decay, half-life, radioactive decay. If x is the number of hours that has elapsed since this experiment began, then the number of bacteria is 100 ( 2) x 6.5. Copy. What is the Formula to Calculate the Exponential Growth?a (or) P 0 0 = Initial amountr = Rate of growthx (or) t = time (time can be in years, days, (or) months, whatever you are using should be consistent throughout the problem) You will notice that in these new growth and decay functions, Exponential decay. The second function is linear. r is the growth rate when r>0 or decay rate when r<0, in percent. 2014-05-22 17:53:33. Exponential Growth: y = a (1 + r)x. Exponential Decay: y = a (1 r)x. Asymptotes are a characteristic of exponential functions. increases by 1, the y value changes by m: In contrast, an exponential function is a function that changes by a constant positive factor. To model population growth and account for carrying capacity and its effect on population, we have to use the equation. Formula of Exponential Growth P (t) = P0 ert Where, t = time (number of periods) P (t) = the amount of some quantity at time t P 0 = initial amount at time t = 0 r = the growth rate e = We will use the EXP function and the exponent (the number in the top right of the equation on the chart with an x at the end) to calculate the growth factor. The word unitary comes from the word unit, which means a single and complete entity. What are the limiting factors to population growth quizlet? Const: It is Best Answer. Answer) Any exponential expression is known as the base and x is known as the exponent. So, from f (0) to f (1), you essentially have to multiply by three. Exponential decay refers to a rapid decrease in a quantity over a period of time.
Question 1124428: Suppose an exponential function f has a 5-unit growth factor of 1.71. a. Choose the y -intercept as one of the two points whenever possible. How do limiting factors affect the carrying capacity of an environment? The following diagram gives the function for exponential growth and decay. N = 3.93 1.34d. An example of an exponential function is the growth of bacteria. When given a percentage of growth or decay, determined the growth/decay factor by adding or subtracting the percent, Explanation: the general formula for any exponential function is ax. Divide 150% by 36 to yield a monthly growth price of 4. And 2P becomes 4P (it doubles itself) in the next 3 years. Final Result. Exponential growth calculator. Exponential decay refers to a rapid decrease in a quantity over a period of time.
m=24* (1.135)*1. 13 answers. An asymptote is a straight line which a curve approaches arbitrarily closely, but never reaches, as it goes to infinity. The equation can be written in the form f (x) = a (1 + r) x or f (x) Exponential growth/decay formula x ( t) = x0 (1 + r) t x (t) is the value at time t. x0 is the initial value at time t=0. As the graph below shows, exponential growth. Growth Factor: (1 + rate of change) is called the growth factor if its value is greater than 1. A function that models exponential growth grows by a rate proportional to the amount present. The variable b represents the growth or decay factor.If b > 1 the function represents exponential growth. An exponential function is a function of the form f (x) = b x, where b > 0 and b 1. The growth factor is 1/6. Section 6.4 Exponential Growth and Decay 313 6.4 EEssential Questionssential Question What are some of the characteristics of exponential growth and exponential decay functions? Remember that the original exponential formula was y = abx. QUESTION. If a quantity is growing at a constant rate of increase, you can model the process with the exponential function y(t) = AB t, where A is the initial amount at time t = 0 and B is the growth factor. a. a is any nonzero number, b. b is a positive real number not equal to 1. (Round your answer to two decimal places.) The exponential curve depends on the exponential function and it depends on the value of the x. Using the exponential growth model I get these $100=Pe^{3r}$ and $400=Pe^{5 Stack Exchange Network Stack Exchange network consists of 180 Q&A communities including
The general rule of thumb is that the exponential growth formula: x (t) = x 0 * (1 + r/100) t is used when there is a quantity with an initial value, x 0, that changes over time, t, with a constant rate of change, r. Therefore , the progress rate can be one hundred percent. In which: x(t) is the number of cases at any given time t x0 is the number of cases at the beginning, also called initial value; b is the number of at first, has a lower rate of growth than the linear equation f(x) =50x; at first, has a slower rate of growth than a cubic function like f(x) = x 3, but Step_2: Press ENTER to apply the formula. f ( x) = abx. Why does the exponential growth show a characteristic J-shaped curve? We can calculate how long it will take to get a billion cells from just one: You can also use the GROWTH worksheet function to fit an exponential An exponential function is defined by the formula f (x) = a x, where the input variable x occurs as an exponent. In this method, we find the value of a unit product from the given number of products, and then we solve for the other number of products. Exponential Function An exponential growth or decay function is a function that grows or shrinks at a constant percent growth rate. Midpoint method example. We will start with an input of 0, and increase each input by 1. GROWTH returns the y-values for a series of new x-values that you specify by using existing x-values and y-values. is the initial or starting value of the function. We will start The decay rate is given in percentage.
An exponential function with growth factor \(2\) eventually grows much more rapidly than a linear function with slope \(2\text{,}\) as you can see by comparing the graphs in the figure or the function values in the tables. 2x,3x) the y -intercept of a graph is the point where it touches the y -axis. The below table shows three different formulas for exponential growth and decay: In the above formulas, the [Math Processing Error] a or [Math Processing Error] P o is the initial f (2) you're gonna multiply by three again. Which graph represents a function with a growth factor of 5? Then set the range of X from one value to another. The Exponential Growth function. We convert it into a decimal by just dropping off % and dividing it by 100. An exponential function can represent growth or decay, and the function that has a growth factor of 5 is f(x) =2(5^x). This calculator has three text fields and two active controls that In financial analysis, GROWTH helps in preparing annual plans or forecasting revenues for a company. It is estimated, that in 1782, there were about 100,000 nesting pairs of bald eagles in the United States. (e.g. Growth is associated with a positive value of {eq}{\color{Red} a} {/eq} in the exponential function, and decay is associated with a negative value of {\color{Red} a} in the exponential function. Where one side of an object matches the other side answers will vary and each angle Find the equation of the line that contains , and , The rigid transformations are translations, The first function is exponential. The exponential function is an important mathematical function which is It's gonna be two, times three squared, which is equal to The of the real exponential function x b x growth of exponential function: Canonical name: GrowthOfExponentialFunction: Date of creation: 2013-03-22 14:51:32: Last (a) What is the yearly growth factor? Definitions: t = time (the time could be the number of days, the number of quarters, the number of years, etc.) Exponential functions have the form f(x) = bx, where b > 0 and b 1. 2+ Ways to Calculate Monthly Growth Rate in Excel. In financial analysis, GROWTH helps in preparing annual plans or forecasting revenues for a Using the growth formula we have y = a (1 + r) x where a = 1 (we start with 1 bacteria), and r = 100%, since the amount doubles. We call b the base or growth factor of f(t): b. Since the investment is in compound interest, for the 4th year, the principal will be 2P. The annual growth rate is $6.07\%$. The function helps calculate predicted exponential growth by using existing data. Predicting a Future Event Work with a partner. On a chart, this curve starts out very slowly, (The formula is valid only up to 1860.) f ( x) = a b x, f ( x) = a b x, where. Exponential growth. Ex. The exponential decay can be used to find food decay, half-life, radioactive decay. Notice the second equation was put in function notation, get used to seeing it both ways! It represents an exponential curve in which the value of Y depends upon the value of X, m is base with X as its exponent, and b are constant. General exponential functions are in the form: y = abx. The equation for "continual" growth (or decay) is A = Pe rt, where "A", is the ending amount, "P" is the beginning amount (principal, in the case of money), "r" is the growth or decay rate We will add 2 This would make the calculation for the straight-line percent change formula (402 - 489) / 489. Described as a function, a quantity undergoing exponential growth is an exponential function of time, that is, the variable representing time is the The fractional change is -5/6; the percent change is - 83 1/3%. 1. What characteristics are used to describe a population? The monthly growth rate is $0.06/12 = 0.005$, so the monthly growth factor is $1.005$. Therefore, at the end of 6 years accumulated value will be 4P. However, for each unit increase in t, t, 2 2 units are added to the value of L(t), L ( t), whereas the value of E(t) E ( t) is multiplied by 2. If. The choices include e x, 10 x or a x. 1164. How do limiting factors affect the carrying capacity of an environment? We will start with an input of 0, and increase each input by 1. Here are some steps to follow when using the exponential or population growth calculator: First, choose the Function from the drop-down menu. (III) Given: the initial value (when x=0) is 21.6. The daily growth factor is $1.005^{1/30} \approx 1.000166265$, so the daily growth rate is about $0.17\%$. The same formula, x(t) Usage Guide Step_1: Write the formula in cell B6. 2. The exponential growth formula is used to find compound interest, find the doubling time, and find the population growth. We will double the corresponding consecutive outputs.
where a stands for the initial amount, b is the growth factor (or in other cases decay factor) and cannot also be = 1 since 1 x power is always 1. How to determine the exponential function?. This answer is: Study guides. In this function, a represents the starting value such as the starting population or the starting dosage level. Why do populations that are not restricted in Exponential growth uses a factor 'r' which is the rate of growth. In this unit, we learn how to construct, analyze, graph, and interpret basic exponential functions of the form f(x)=ab. Just as in any exponential expression, b is called the base and x is called the exponent. Let `y=f(x)=ab^x` represents the general form of the exponential function, When a`>0` and b`>0` , b represents the growth factor, When x=0 , then y=a which is the y-intercept. What is the 2-unit growth factor for f? Remember that our original exponential formula is equal to y = ab x.You will notice that in the Finally, input the value of the increment. Lets assume the CEO expects annual revenues to reach $1,500,000. Using the basic formula for exponential growth f ( x) = a ( 1 + r) x we can write the formula, f ( t) = 1.14 ( 1 + 0.0134) t. To It is also referred to as the Decay Calculator. Decay Factor: (1 + rate of change) is called the decay factor if Exponential decay is more or less the same as exponential growth, except that the exponential function decreases over time rather than increases. ), and this calculator will estimate the appropriate exponential function and will provide its graph. Definitions: t = time (the time could be the number of days, the number of quarters, the number of years, etc.) For example, if a population starts out at 1000 individuals and grows by 5% each year, the equation is y(t) = 1000(1.05) t. The exponential function. Wiki User. It occurs when the instantaneous rate of change (that is, the derivative) of a quantity with respect to time is proportional to the quantity itself. We will double the corresponding consecutive outputs. Finally you take your growth factor as a percentage and change it into a decimal before plugging it into y = a(b)x. where. Increasing demand for disruptive battery management systems will enable the battery management system market exhibit above 15% Y-o-Y growth in 2021The U.S. The U.K. Germany and France will continue exhibiting high demand for battery management systemsSouth Korea and Japan will lucrative markets for BMS in East Asia (IV) F(x) = 21.6(1/6)^x Note: Use the fractional form of the growth factor because it is exact; a decimal would only be an approximation. y = 1 (1 + 1.00) x = 2 x.
